Steiner's Porism
(There is also a
german version of this page.)
What is it about?
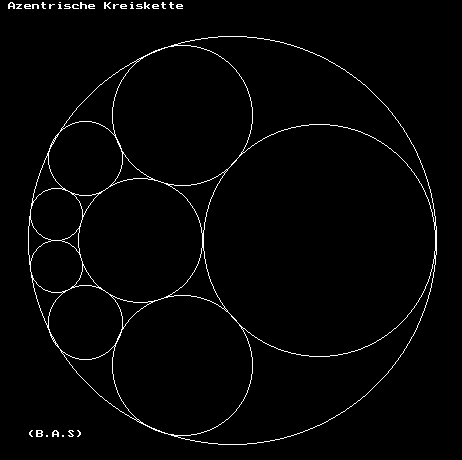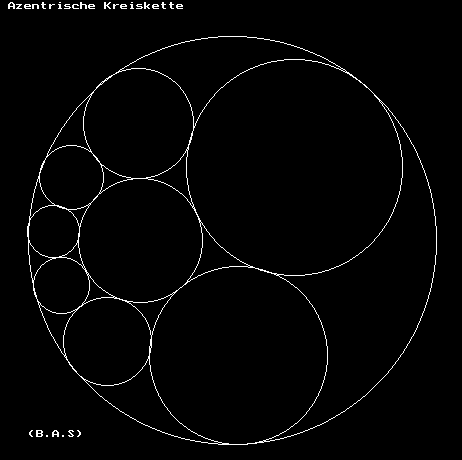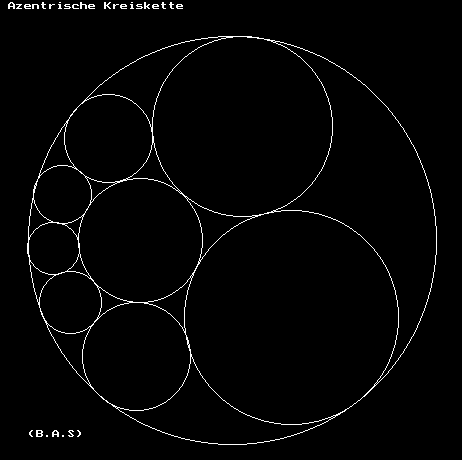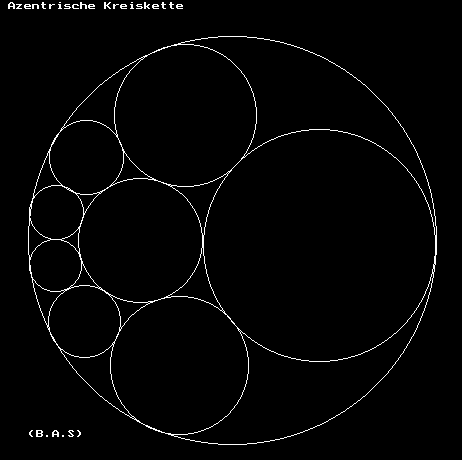
In a course for high school students in the winter term 2000/01 entitled Propädeutikum Mathematik, we studied Steiner's beautiful result on closed chains of circles. The statement of this theorem is quite surprising:
If there is a closed chain of circles that is tangent to two given circles, then there are infinitely many such chains.
Can we see this theorem »in action«?
My colleague B. Schmitt programmed a nice animation, which you can see below (if your browser displays animations). The inner circle and the outer circle are chosen in such a way that there exists a closed chain of circles in between them. Steiner' theorem guarantees then that we may »rotate« this chain.Did you notice that the circles continually change their radius when they move around? Nonetheless we can be sure that the chain remains closed at any time; it will never break apart -- that is what Steiner's result essentially says.